Understanding the distance required to escape Earth’s gravitational field involves grasping the concept of gravity wells. A gravity well is a metaphor describing the energy needed to overcome a celestial body’s gravitational pull. This provides a straightforward way to address the question of how far must one travel to escape earth’s gravitational field.
There are two primary ways to escape a gravity well, like Earth’s. One is to achieve a high velocity over a short distance, the method used to launch rockets from Earth. The other involves a gradual climb over a longer distance, suitable when gravitational forces are weaker. Both approaches involve adding energy, typically through fuel, to ascend the “side” of the gravity well. This energy becomes potential energy as you move away from the surface. Reaching escape velocity means your potential energy overcomes the gravitational pull, allowing you to move straight ahead, no longer bound by the well’s curve.
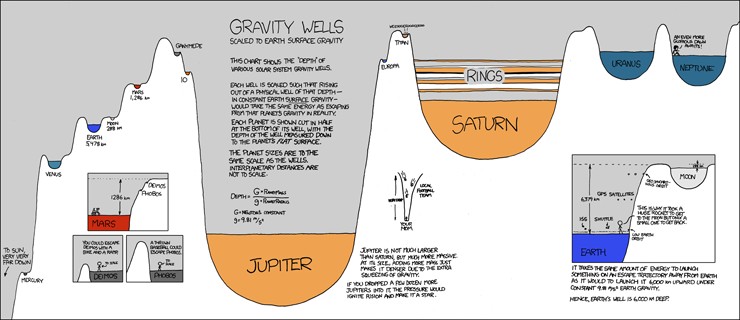Illustration of a gravity well, showing the energy required to escape.
Failing to climb far enough to maintain sufficient velocity will result in falling back towards the body, as escape velocity was not achieved.
At any point in space, multiple gravitational forces exist. However, one will exert a stronger influence than the others, defined by the sphere of influence. Near Earth, including low Earth orbit, Earth’s gravity dominates. Traveling towards the Moon, lunar gravity becomes dominant after passing the Earth-Moon system L1 Lagrangian point.
The “depth” of a gravity well is often expressed as its escape velocity at the bottom of the well. Earth’s gravity well depth is approximately 11.2 km/s, the escape velocity at its surface. While Wikipedia notes an escape velocity of 7.1 km/s at 9,000 km above Earth, reaching that altitude requires significant energy, offsetting the benefit of a lower absolute speed needed to break free.
Ultimately, escaping Earth’s gravity isn’t just about distance; it’s about achieving sufficient velocity to overcome the gravitational pull at a given point. The further you travel, the less velocity you need.
